Giải bài 79 trang 108 SBT toán 10 - Cánh diều

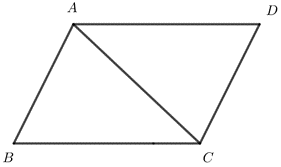
Đề bài

a) Chứng minh đẳng thức \({\left| {\overrightarrow a  + \overrightarrow b } \right|^2} = {\left| {\overrightarrow a } \right|^2} + {\left| {\overrightarrow b } \right|^2} + 2\overrightarrow a .\overrightarrow b \) với \(\overrightarrow a ,\overrightarrow b \) là hai vectơ bất kì

b) Cho \(\left| {\overrightarrow a } \right| = 2,\left| {\overrightarrow b } \right| = 3,\left| {\overrightarrow a  + \overrightarrow b } \right| = \sqrt 7 \). Tinh \(\overrightarrow a .\overrightarrow b \) và \(\left( {\overrightarrow a ,\overrightarrow b } \right)\)

Lời giải chi tiết

a) Xét hình bình hành ABCD thỏa mãn \(\overrightarrow {AB}  = \overrightarrow a ,\overrightarrow {AD}  = \overrightarrow b \)

Theo quy tắc hình bình hành ta có:

 \(\overrightarrow a  + \overrightarrow b  = \overrightarrow {AB}  + \overrightarrow {AD}  = \overrightarrow {AC}  \Rightarrow \left| {\overrightarrow a  + \overrightarrow b } \right| = AC\)

Mà \(A{C^2} = A{B^2} + B{C^2} - 2AB.AC.\cos B = A{B^2} + A{D^2} - 2AB.AD.\cos B\)

Mặt khác, \(\widehat {BAD} + \widehat B = {180^0} \Rightarrow \cos \widehat B =  - \cos \widehat {BAD}\)

\( \Rightarrow A{C^2} = A{B^2} + A{D^2} + 2AB.AD.\cos \widehat {BAD} = {\left| {\overrightarrow {AB} } \right|^2} + {\left| {\overrightarrow {AD} } \right|^2} + 2AB.AD.\cos \left( {\overrightarrow {AB} ,\overrightarrow {AD} } \right) = {\left| {\overrightarrow {AB} } \right|^2} + {\left| {\overrightarrow {AD} } \right|^2} + 2\overrightarrow {AB} .\overrightarrow {AD} \)

\( \Rightarrow {\left| {\overrightarrow a  + \overrightarrow b } \right|^2} = {\left| {\overrightarrow a } \right|^2} + {\left| {\overrightarrow b } \right|^2} + 2\overrightarrow a .\overrightarrow b \) (ĐPCM)

b) Theo a) \({\left| {\overrightarrow a  + \overrightarrow b } \right|^2} = {\left| {\overrightarrow a } \right|^2} + {\left| {\overrightarrow b } \right|^2} + 2\overrightarrow a .\overrightarrow b \)

\( \Rightarrow \overrightarrow a .\overrightarrow b  = \frac{{{{\left| {\overrightarrow a  + \overrightarrow b } \right|}^2} - {{\left| {\overrightarrow a } \right|}^2} - {{\left| {\overrightarrow b } \right|}^2}}}{2} = \frac{{{{\sqrt 7 }^2} - {2^2} - {3^2}}}{2} =  - 3\)

Ta có: \(\overrightarrow a .\overrightarrow b  =  - 3 \Leftrightarrow \left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|.\cos \left( {\overrightarrow a ,\overrightarrow b } \right) =  - 3 \Leftrightarrow \cos \left( {\overrightarrow a ,\overrightarrow b } \right) = \frac{{ - 3}}{{\left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|}} =  - \frac{1}{2}\) \( \Rightarrow \left( {\overrightarrow a ,\overrightarrow b } \right) = {120^0}\)