Bài tập 12 trang 90 Tài liệu dạy – học Toán 8 tập 2Giải bài tập a) Hình a, cho biết Quảng cáo
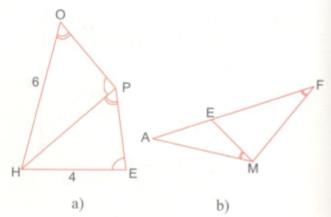
Đề bài a) Hình a, cho biết \(\widehat O = \widehat {HPE};\,\,\widehat {HPO} = \widehat E;\,\,\,OH = 6;\)\(\,\,HE = 4.\) Tính HP. b) Hình b, cho biết \(\widehat {AME} = \widehat {AFM.}\) Chứng minh: AM2 = AE.AF. Lời giải chi tiết a) Xét hai tam giác OHP và HPE có: \(\widehat O = \widehat {HPE}(gt)\) và \(\widehat {HPO} = \widehat E(gt)\) Do đó \(\Delta OHP \sim \Delta PHE(g.g)\) \(\Rightarrow {{OH} \over {PH}} = {{HP} \over {HE}}\) \(\Rightarrow {6 \over {HP}} = {{HP} \over 4}\) \(\Rightarrow H{P^2} = 24 \Rightarrow HP = 2\sqrt 6 \) b) Xét ∆AME và ∆AFM ta có: \(\widehat {AME} = \widehat {AFM}(gt)\) và \(\widehat {EAM}\) chung \( \Rightarrow \Delta AME \sim \Delta AFM(g.g)\) \(\Rightarrow {{AM} \over {AF}} = {{AE} \over {AM}}\) \(\Rightarrow A{M^2} = AE.AF\) Loigiaihay.com  Chia sẻ Bình luận
Quảng cáo
Tham Gia Group Dành Cho 2K11 Chia Sẻ, Trao Đổi Tài Liệu Miễn Phí
|
