Đầy đủ tất cả các môn
Bài 3 trang 94 SGK Đại số 10Giải các bất phương trình... Quảng cáo
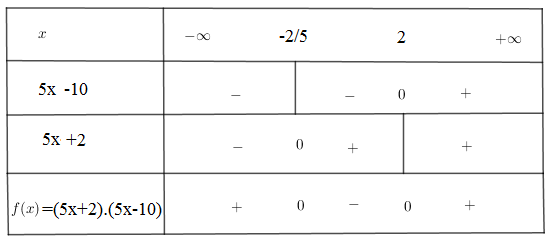
Video hướng dẫn giải Giải các bất phương trình LG a |5x−4|≥6|5x−4|≥6; Phương pháp giải: |f(x)|≤a⇔−a≤f(x)≤a|f(x)|≥a⇔[f(x)≤−af(x)≥a(a>0) Lời giải chi tiết: |5x−4|≥6 Cách 1: ⇔(5x−4)2≥62⇔(5x−4)2−62≥0⇔(5x−4−6)(5x−4+6)≥0⇔(5x−10)(5x+2)≥0 Bảng xét dấu: Ta có: 5x−10=0⇔x=25x+2=0⇔x=−25
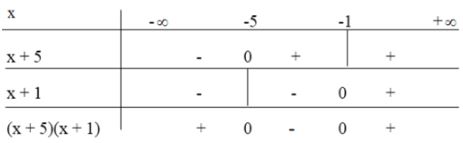
Từ bảng xét dấu cho tập nghiệm của bất phương trình: T=(−∞;−25]∪[2;+∞). Cách 2: |5x−4|≥6⇔[5x−4≥65x−4≤−6⇔[5x≥105x≤−2⇔[x≥2x≤−25 Vậy tập nghiệm của bất phương trình: T=(−∞;−25]∪[2;+∞). LG b |−5x+2|<|10x−1|. Lời giải chi tiết: |−5x+2|<|10x−1| Cách 1: ⇔5|x+2|<10|x−1|(DK:x≠−2,x≠1)⇔1|x+2|<2|x−1|⇔2|x+2|−|x−1|>0 (1) Bảng xét dấu: +) Với x<−2 ta có: (1)⇔2(−x−2)−(−x+1)>0⇔−2x−4+x−1>0⇔−x−5>0⇔−x>5⇔x<−5 Kết hợp với x<−2 ta được x<−5. +) Với −2<x<1 ta có: (1)⇔2(x+2)−(−x+1)>0⇔2x+4+x−1>0⇔3x+3>0⇔3x>−3⇔x>−1 Kết hợp với −2<x<1 ta được −1<x<1 +) Với x>1 ta có: (1)⇔2(x+2)−(x−1)>0⇔2x+4−x+1>0⇔x+5>0⇔x>−5 Kết hợp với x>1 ta được x>1. Vậy bất phương trình có nghiệm [x<−5−1<x<1x>1 Tập nghiệm của bất phương trình là: T=(−∞;−5)∪(−1;1)∪(1;+∞). Cách 2: 5|x+2|<10|x−1|(DK:x≠−2,x≠1)⇔1|x+2|<2|x−1|⇒|x−1|<2|x+2|⇔(x−1)2<4(x+2)2⇔x2−2x+1<4(x2+4x+4)⇔3x2+18x+15>0⇔3(x+1)(x+5)>0 Ta có bảng xét dấu: Xét dấu ta được [x<−5x>−1 Kết hợp điều kiện x≠−2,x≠1 ta được tập nghiệm của bất phương trình là: T=(−∞;−5)∪(−1;1)∪(1;+∞). Loigiaihay.com
Quảng cáo
|
























 Tải app
Tải app



