Giải bài 4.19 trang 54 sách bài tập toán 10 - Kết nối tri thức với cuộc sốngCho tam giác ABC Tổng hợp đề thi học kì 1 lớp 10 tất cả các môn - Kết nối tri thức Toán - Văn - Anh - Lí - Hóa - Sinh - Sử - Địa... Quảng cáo
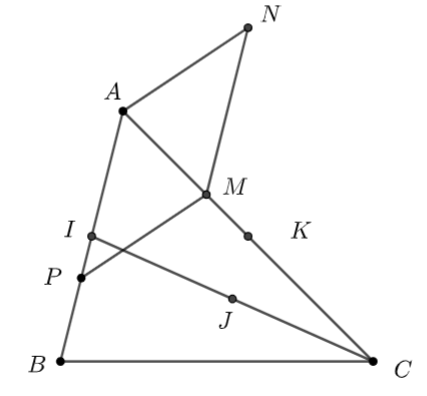
Đề bài Cho tam giác \(ABC.\) a) Tìm điểm \(M\) sao cho \(\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} = \overrightarrow 0 \) b) Xác định điểm \(N\) thỏa mãn \(4\overrightarrow {NA} - 2\overrightarrow {NB} + \overrightarrow {NC} = \overrightarrow 0 \) Lời giải chi tiết
a) Giả sử tìm được điểm \(M\) sao cho \(\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} = \overrightarrow 0 \) Gọi \(I\) là trung điểm của \(AB\) và \(J\) là trung điểm của cạnh \(CI\). Ta có: \(\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} = \;\overrightarrow {MI} + \overrightarrow {IA} + \overrightarrow {MI} + \overrightarrow {IB} + 2\overrightarrow {MC} = 2\overrightarrow {MI} + 2\overrightarrow {MC} = 4\overrightarrow {MJ} \) Mặt khác \(\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} = \overrightarrow 0 \) \( \Rightarrow \) \(4\overrightarrow {MJ} = \overrightarrow 0 \,\, \Rightarrow \,\,\overrightarrow {MJ} = \overrightarrow 0 \,\, \Rightarrow \,\,M \equiv J\) Vậy \(M\) là trung điểm của \(CI\). b) Giả sử tìm được điểm \(N\) thỏa mãn \(4\overrightarrow {NA} - 2\overrightarrow {NB} + \overrightarrow {NC} = \overrightarrow 0 \) Gọi \(K\) là trung điểm của \(AC\). Ta có: \(4\overrightarrow {NA} - 2\overrightarrow {NB} + \overrightarrow {NC} = 2\left( {\overrightarrow {NA} - \overrightarrow {NB} } \right) + \left( {\overrightarrow {NA} + \overrightarrow {NC} } \right) + \overrightarrow {NA} \) \(\begin{array}{l} = 2\overrightarrow {BA} + \left( {\overrightarrow {NK} + \overrightarrow {KB} + \overrightarrow {NK} + \overrightarrow {KC} } \right) + \overrightarrow {NA} \\ = 2\overrightarrow {BA} + 2\overrightarrow {NK} + \overrightarrow {NA} \end{array}\) Gọi \(M\) là điểm thỏa mãn \(2\overrightarrow {MK} + \overrightarrow {MA} = 0\) Khi đó: \(2\overrightarrow {NK} + \overrightarrow {NA} = 2\left( {\overrightarrow {NM} + \overrightarrow {MK} } \right) + \overrightarrow {NM} + \overrightarrow {MA} = 3\overrightarrow {NM} \) Do đó \(4\overrightarrow {NA} - 2\overrightarrow {NB} + \overrightarrow {NC} = 2\overrightarrow {BA} + 3\overrightarrow {NM} \) Mặt khác \(4\overrightarrow {NA} - 2\overrightarrow {NB} + \overrightarrow {NC} = \overrightarrow 0 \) \( \Rightarrow \) \(2\overrightarrow {BA} + 3\overrightarrow {NM} = \overrightarrow 0 \) \( \Leftrightarrow \) \(\overrightarrow {NM} = \frac{2}{3}\overrightarrow {AB} \) (1) Lấy điểm \(P\) thuộc cạnh \(AB\) sao cho \(\overrightarrow {AP} = \frac{2}{3}\overrightarrow {AB} \) (2) Từ (1) và (2) \( \Rightarrow \) \(\overrightarrow {NM} = \overrightarrow {AP} \) \( \Rightarrow \) tứ giác \(APMN\) là hình bình hành Vậy điểm \(N\) cần tìm là đỉnh thứ tư của hình bình hành \(APMN\).
Quảng cáo
|