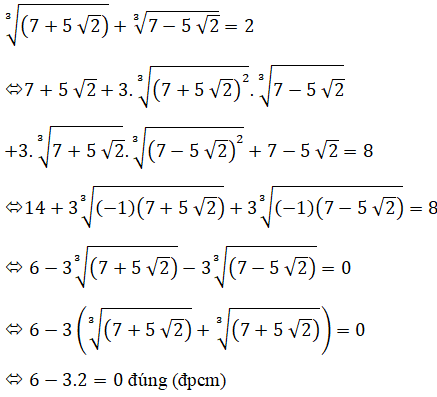Bài 7 trang 76 SGK Đại số và Giải tích 12 Nâng cao

Đề bài

Chứng minh \(\root 3 \of {7 + 5\sqrt 2 }  + \root 3 \of {7 - 5\sqrt 2 }  = 2\)

Lời giải chi tiết

Đặt \(x = \root 3 \of {7 + 5\sqrt 2 }  + \root 3 \of {7 - 5\sqrt 2 } \)

Ta có:

\({x^3} = \left( {\root 3 \of {7 + 5\sqrt 2 }  + \root 3 \of {7 - 5\sqrt 2 } } \right)^3\)

\( = 7 + 5\sqrt 2  + 7 - 5\sqrt 2  \) \(+ 3\root 3 \of {{{\left( {7 + 5\sqrt 2 } \right)}^2}} .\root 3 \of {7 - 5\sqrt 2 }  \) \(+ 3\root 3 \of {7 + 5\sqrt 2 } .\root 3 \of {{{\left( {7 - 5\sqrt 2 } \right)}^2}} \)

\(= 14 + 3\sqrt[3]{{\left( {7 + 5\sqrt 2 } \right)\left( {7 - 5\sqrt 2 } \right)}}.\)\(.\left( {\sqrt[3]{{7 + 5\sqrt 2 }} + \sqrt[3]{{7 - 5\sqrt 2 }}} \right)\)

\( = 14 - 3\left( {\root 3 \of {7 + 5\sqrt 2 }  + \root 3 \of {7 - 5\sqrt 2 } } \right) \)

\(= 14 - 3x\).

Từ đó suy ra: \({x^3} + 3x - 14 = 0\,\,\,\,\left( 1 \right)\)

\(\left( 1 \right) \Leftrightarrow \left( {x - 2} \right)\left( {{x^2} + 2x + 7} \right) = 0 \)

\(\Leftrightarrow x - 2 = 0 \Leftrightarrow x = 2\)

(vì \({x^2} + 2x + 7 > 0\))

Vậy \(\root 3 \of {7 + 5\sqrt 2 }  + \root 3 \of {7 - 5\sqrt 2 }  = 2\)

Cách khác:

Loigiaihay.com