Câu hỏi:
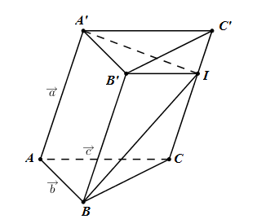
Cho lăng trụ tam giác ABC.A’B’C’. Đặt \(AA' = a;\,\,AB = b,\,\,AC = c\). Gọi I là điểm thuộc đường thẳng CC’ sao cho \(\overrightarrow {C'I} = \dfrac{1}{3}\overrightarrow {C'C} \), G là điểm thỏa mãn \(\overrightarrow {GA} + \overrightarrow {GB} + \overrightarrow {GC} + \overrightarrow {GD} = \overrightarrow 0 \) . Biểu diễn vectơ\(\overrightarrow {IG} \) qua các vectơ \(\overrightarrow a ;\,\,\overrightarrow b ;\,\,\overrightarrow c \). Trong các khẳng định sau, khẳng định nào là khẳng định đúng?
- A \(\overrightarrow {IG} = \dfrac{1}{4}\left( {\dfrac{1}{3}\overrightarrow a + 2\overrightarrow b - 3\overrightarrow c } \right)\)
- B \(\overrightarrow {IG} = \dfrac{1}{3}\left( {\overrightarrow a + \overrightarrow b + 2\overrightarrow c } \right)\)
- C \(\overrightarrow {IG} = \dfrac{1}{4}\left( {\overrightarrow a + \overrightarrow c - 2\overrightarrow b } \right)\)
- D \(\overrightarrow {IG} = \dfrac{1}{4}\left( {\overrightarrow b + \dfrac{1}{3}\overrightarrow c - 2\overrightarrow a } \right)\)
Phương pháp giải:
Sử dụng công thức ba điểm.
Lời giải chi tiết:
\(\begin{array}{l}\overrightarrow {GB} + \overrightarrow {GA'} + \overrightarrow {GB'} + \overrightarrow {GC'} = \overrightarrow 0 \\ \Leftrightarrow \overrightarrow {GI} + \overrightarrow {IB} + \overrightarrow {GI} + \overrightarrow {IA'} + \overrightarrow {GI} + \overrightarrow {IB'} + \overrightarrow {GI} + \overrightarrow {IC'} = \overrightarrow 0 \\ \Leftrightarrow 4\overrightarrow {GI} + \left( {\overrightarrow {IB} + \overrightarrow {IA'} + \overrightarrow {IB'} + \overrightarrow {IC'} } \right) = \overrightarrow 0 \\ \Leftrightarrow \overrightarrow {IG} = \dfrac{1}{4}\left( {\overrightarrow {IC} + \overrightarrow {CA} + \overrightarrow {AB} + \overrightarrow {IC'} + \overrightarrow {C'A'} + \overrightarrow {IC'} + \overrightarrow {C'A'} + \overrightarrow {A'B'} + \overrightarrow {IC'} } \right)\\ \Leftrightarrow \overrightarrow {IG} = \dfrac{1}{4}\left( {\overrightarrow {IC} + 3\overrightarrow {IC'} + 3\overrightarrow {CA} + 2\overrightarrow {A'B'} } \right)\\ \Leftrightarrow \overrightarrow {IG} = \dfrac{1}{4}\left( { - \dfrac{2}{3}\overrightarrow a + \overrightarrow a - 3\overrightarrow c + 2\overrightarrow b } \right) = \dfrac{1}{4}\left( {\dfrac{1}{3}\overrightarrow a + 2\overrightarrow b - 3\overrightarrow c } \right)\end{array}\)
Chọn A.
Câu hỏi trước
