Giải đề thi học kì 2 toán lớp 10 năm 2020 - 2021 trường THPT Nguyễn Xuân ÔnLàm bàiQuảng cáo
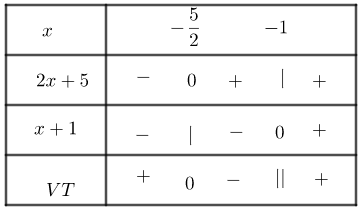
Câu hỏi 1 : Giải bất phương trình: Câu 1: \(\dfrac{3}{{x + 1}} \ge - 2\) Phương pháp giải: Chuyển vế rồi quy đồng. Sử dụng dấu của nhị thức bậc nhất. Lời giải chi tiết: \(\begin{array}{l}\dfrac{3}{{x + 1}} \ge - 2 \Leftrightarrow \dfrac{3}{{x + 1}} + 2 \ge 0\\ \Leftrightarrow \dfrac{{2x + 5}}{{x + 1}} \ge 0\end{array}\) Bảng xét dấu:
Từ bảng xét dấu ta thấy \(\dfrac{{2x + 5}}{{x + 1}} \ge 0 \Leftrightarrow \left[ \begin{array}{l}x \le - \dfrac{5}{2}\\x > - 1\end{array} \right.\) Câu 2: \(\sqrt {{x^2} - 10x + 9} \le 2x - 3\) Phương pháp giải: \(\sqrt {f\left( x \right)} \le g\left( x \right) \Leftrightarrow \left\{ \begin{array}{l}g\left( x \right) \ge 0\\f\left( x \right) \ge 0\\f\left( x \right) \le {g^2}\left( x \right)\end{array} \right.\) Lời giải chi tiết: \(\begin{array}{l}\sqrt {{x^2} - 10x + 9} \le 2x - 3\\ \Leftrightarrow \left\{ \begin{array}{l}{x^2} - 10x + 9 \ge 0\\2x - 3 \ge 0\\{x^2} - 10x + 9 \le {\left( {2x - 3} \right)^2}\end{array} \right.\\ \Leftrightarrow \left\{ \begin{array}{l}\left[ \begin{array}{l}x \ge 9\\x \le 1\end{array} \right.\\x \ge \dfrac{3}{2}\\3{x^2} - 2x \ge 0\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}x \ge 9\\\left[ \begin{array}{l}x \ge \dfrac{3}{2}\\x \le 0\end{array} \right.\end{array} \right.\\ \Leftrightarrow x \ge 9\end{array}\) Vậy \(S = \left[ {9; + \infty } \right)\) Câu hỏi 2 : Tìm m để phương trình: \(\left( {m + 1} \right){x^2} + 2\left( {m + 2} \right)x + 3m + 4 = 0\) có hai nghiệm dương phân biệt. Phương pháp giải: Phương trình \(a{x^2} + bx + c = 0\) có 2 nghiệm dương phân biệt\( \Leftrightarrow \left\{ \begin{array}{l}a \ne 0\\\Delta > 0\\S = - \dfrac{a}{b} > 0\\P = \dfrac{c}{a} > 0\end{array} \right.\) Lời giải chi tiết: \(\left( {m + 1} \right){x^2} + 2\left( {m + 2} \right)x + 3m + 4 = 0\) có hai nghiệm dương phân biệt. \(\begin{array}{l} \Leftrightarrow \left\{ \begin{array}{l}m + 1 \ne 0\\{\left( {m + 2} \right)^2} - \left( {m + 1} \right).\left( {3m + 4} \right) > 0\\\dfrac{{ - 2\left( {m + 2} \right)}}{{m + 1}} > 0\\\dfrac{{3m + 4}}{{m + 1}} > 0\end{array} \right.\\ \Leftrightarrow \left\{ \begin{array}{l}m \ne - 1\\ - 2{m^2} - 3m > 0\\ - 2 < m < - 1\\\left[ \begin{array}{l}m > - 1\\m < - \dfrac{4}{3}\end{array} \right.\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l} - \dfrac{3}{2} < m < 0\\ - 2 < m < - 1\\m < - \dfrac{4}{3}\end{array} \right.\\ \Leftrightarrow - \dfrac{3}{2} < m < - \dfrac{4}{3}\end{array}\) Câu hỏi 3 : Tính Câu 1: Cho \(\sin \alpha = \dfrac{1}{3}\) và \(\dfrac{\pi }{2} < \alpha < \pi \). Tính \(\cos \alpha ;\sin \left( {\alpha + \dfrac{\pi }{6}} \right)\) và \(\cos 2\alpha \). Phương pháp giải: Sử dụng công thức: \({\sin ^2}x + {\cos ^2}x = 1\) Xác định dấu của \(\cos x\) Lời giải chi tiết: \(\begin{array}{l}{\sin ^2}x + {\cos ^2}x = 1\\ \Rightarrow \left| {\cos x} \right| = \sqrt {1 - \dfrac{1}{9}} = \dfrac{{2\sqrt 2 }}{3}\\\dfrac{\pi }{2} < \alpha < \pi \Rightarrow \cos x < 0\\ \Rightarrow \cos x = - \dfrac{{2\sqrt 2 }}{3}\end{array}\) \(\begin{array}{l}\sin \left( {\alpha + \dfrac{\pi }{6}} \right)\\ = \sin \alpha .\cos \dfrac{\pi }{6} + \cos \alpha .\sin \dfrac{\pi }{6}\\ = \dfrac{1}{3}.\dfrac{{\sqrt 3 }}{2} + \dfrac{{2\sqrt 2 }}{3}.\dfrac{1}{2}\\ = \dfrac{{\sqrt 3 + 2\sqrt 2 }}{6}\end{array}\) \(\cos 2\alpha = 2{\cos ^2}x - 1 = 2.\dfrac{8}{9} - 1 = \dfrac{7}{9}\) Câu 2: Chứng minh đẳng thức sau: \(\dfrac{{\cos 2x + 2{{\sin }^2}x}}{{1 - \cos 2x + \sin 2x}}.\left( {1 + \tan x} \right) = \dfrac{1}{{\sin 2x}}\) Phương pháp giải: Biến đổi vế trái về vế phải. \(\cos 2x = 1 - 2{\sin ^2}x\). Lời giải chi tiết: \(\begin{array}{l}VT = \dfrac{{\cos 2x + 2{{\sin }^2}x}}{{1 - \cos 2x + \sin 2x}}.\left( {1 + \tan x} \right)\\ = \dfrac{1}{{2{{\sin }^2}x + \sin 2x}}.\dfrac{{\sin x + \cos x}}{{\cos x}}\\ = \dfrac{1}{{2\sin x\left( {\sin x + \cos x} \right)}}.\dfrac{{\sin x + \cos x}}{{\cos x}}\\ = \dfrac{1}{{2\sin x.\cos x}} = \dfrac{1}{{\sin 2x}} = VP\end{array}\) Câu hỏi 4 : Trong mặt phẳng tọa độ Oxy, cho đường tròn (C): \({x^2} + {y^2} + 6x - 4y + 9 = 0\) và đường thẳng \(\Delta :3x - 4y + 2 = 0\). Câu 1: Tìm tâm và bán kính của đường tròn (C). Phương pháp giải: Đưa phương trình đường tròn về đạng chính tắc: \({\left( {x - {x_0}} \right)^2} + {\left( {y - {y_0}} \right)^2} = {R^2}\) Lời giải chi tiết: \(\begin{array}{l}{x^2} + {y^2} + 6x - 4y + 9 = 0\\ \Leftrightarrow {\left( {x + 3} \right)^2} + {\left( {y - 2} \right)^2} = {3^2} + {2^2} - 9\\ \Leftrightarrow {\left( {x + 3} \right)^2} + {\left( {y - 2} \right)^2} = {2^2}\end{array}\) Vậy (C) có tâm I(-3;2), bán kính R=2. Câu 2: Viết phương trình tiếp tuyến của đường tròn (C) biết tiếp tuyến vuông góc với đường thẳng \(\Delta \). Phương pháp giải: \(\Delta '\) là tiếp tuyến của đường tròn (C)\( \Leftrightarrow d\left( {I,\Delta '} \right) = R\) với I là tâm và R là bán kính đường tròn. \(\Delta '\) vuông góc với đường thẳng \(\Delta :ax + by + c = 0\) thì nhận \(\overrightarrow n \left( {b; - a} \right)\) làm vecto pháp tuyến. Lời giải chi tiết: Gọi \(\Delta '\) là tiếp tuyến cần tìm. khi đó \(\Delta ' \bot \Delta \) nên có dạng: \(4x + 3y + c = 0\). Vì \(\Delta '\) là tiếp tuyến của đường tròn (C) nên \(\begin{array}{l}d\left( {I,\Delta '} \right) = 2\\ \Leftrightarrow \dfrac{{\left| {4.\left( { - 3} \right) + 3.2 + c} \right|}}{5} = 2\\ \Leftrightarrow \left| {c - 6} \right| = 10 \Leftrightarrow \left[ \begin{array}{l}c = 16\\c = - 4\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}\Delta ':4x + 3y + 16 = 0\\\Delta ':4x + 3y - 4 = 0\end{array} \right.\end{array}\) Câu 3: Viết phương trình đường thẳng (d) đi qua điểm \(M\left( { - 2; - 1} \right)\) và cắt đường tròn (C) tại hai điểm A, B sao cho \(AB = 2\sqrt 3 \). Phương pháp giải: Gọi phương trình đường thẳng đi qua điểm \(M\left( {{x_0};{y_0}} \right)\) là \(a\left( {x - {x_0}} \right) + b\left( {y - {y_0}} \right) = 0\). (d) cắt đường tròn (C) tại hai điểm A, B thì \({\left[ {d\left( {I,d} \right)} \right]^2} + {\left( {\dfrac{{AB}}{2}} \right)^2} = {R^2}\) theo Py- ta- go. Lời giải chi tiết: Gọi phương trình đường thẳng đi qua điểm \(M\left( { - 2; - 1} \right)\) là \(a\left( {x + 2} \right) + b\left( {y + 1} \right) = 0\) \( \Leftrightarrow ax + by + 2a + b = 0\). (d) cắt đường tròn (C) tại hai điểm A, B nên \({\left[ {d\left( {I,d} \right)} \right]^2} + {\left( {\dfrac{{AB}}{2}} \right)^2} = {R^2}\) \(\begin{array}{l} \Leftrightarrow d\left( {I,d} \right) = 1\\ \Leftrightarrow \dfrac{{\left| {a.\left( { - 3} \right) + b.2 + 2a + b} \right|}}{{\sqrt {{a^2} + {b^2}} }} = 1\\ \Leftrightarrow \left| { - a + 3b} \right| = \sqrt {{a^2} + {b^2}} \\ \Leftrightarrow {a^2} - 6ab + 9{b^2} = {a^2} + {b^2}\\ \Leftrightarrow - 6ab + 8{b^2} = 0 \Leftrightarrow \left[ \begin{array}{l}b = 0\\3a = 4b\end{array} \right.\end{array}\) Với \(b = 0 \Rightarrow \left( d \right):x + 2 = 0\) Với \(3a = 4b\). Chọn a=4,b=3. Khi đó \(\left( d \right):4x + 3y + 11 = 0\) Vậy \(\left( d \right):x + 2 = 0\) hoặc \(\left( d \right):4x + 3y + 11 = 0\) Câu hỏi 5 : Giải bất phương trình: \(3x + 1 + \sqrt {9{x^2} - 12x + 1} \ge 3\sqrt {3x} \) Phương pháp giải: Tìm điều kiện xác định của bất phương trình: \(\sqrt {f\left( x \right)} \) xác định \( \Leftrightarrow f\left( x \right) \ge 0\) Lời giải chi tiết: Điều kiện: \(\left\{ \begin{array}{l}9{x^2} - 12x + 1 \ge 0\\x \ge 0\end{array} \right. \Leftrightarrow \left[ \begin{array}{l}x \ge 2 + \sqrt 3 \\0 \le x \le 2 - \sqrt 3 \end{array} \right.\) \(3x + 1 + \sqrt {9{x^2} - 12x + 1} \ge 3\sqrt {3x} \) \( \Leftrightarrow 6x + 2 + 2\sqrt {9{x^2} - 12x + 1} \)\( - 6\sqrt {3x} \ge 0\) \( \Leftrightarrow 6x - 5\sqrt {3x} + 2\)\( + \left( {2\sqrt {9{x^2} - 12x + 1} - \sqrt {3x} } \right) \ge 0\) \( \Leftrightarrow 2.{\left( {\sqrt {3x} } \right)^2} - 5.\sqrt {3x} + 2\)\( + \dfrac{{36{x^2} - 51x + 4}}{{2\sqrt {9{x^2} - 12x + 1} + \sqrt {3x} }} \ge 0\) \( \Leftrightarrow \left( {\sqrt {3x} - 2} \right)\left( {2\sqrt {3x} - 1} \right)\)\( + \dfrac{{\left( {3x - 4} \right)\left( {12x - 1} \right)}}{{2\sqrt {9{x^2} - 12x + 1} + \sqrt {3x} }} \ge 0\) \( \Leftrightarrow \dfrac{{\left( {3x - 4} \right)\left( {12x - 1} \right)}}{{\left( {\sqrt {3x} + 2} \right)\left( {2\sqrt {3x} + 1} \right)}}\)\( + \dfrac{{\left( {3x - 4} \right)\left( {12x - 1} \right)}}{{2\sqrt {9{x^2} - 12x + 1} + \sqrt {3x} }} \ge 0\) \( \Leftrightarrow \left( {3x - 4} \right)\left( {12x - 1} \right) \ge 0\) (1) Vì \(\dfrac{1}{{\left( {\sqrt {3x} + 2} \right)\left( {2\sqrt {3x} + 1} \right)}} + \dfrac{1}{{2\sqrt {9{x^2} - 12x + 1} + \sqrt {3x} }} > 0\) (1)\( \Leftrightarrow \left[ \begin{array}{l}x \ge \dfrac{4}{3}\\x \le \dfrac{1}{{12}}\end{array} \right.\)kết hơp với điều kiện ta được: \(\left[ \begin{array}{l}x \ge 2 + \sqrt 3 \\0 \le x \le \dfrac{1}{{12}}\end{array} \right.\) Vậy \(S = \left[ {0;\dfrac{1}{{12}}} \right] \cup \left[ {2 + \sqrt 3 ; + \infty } \right)\). Quảng cáo
|