Câu hỏi:
Giải các bất phương trình sau:
Câu 1:
x−1x2+4x+3≥0
Phương pháp giải:
Giải x2+4x+3=0.
Lập bảng xét dấu.
Lời giải chi tiết:
x−1x2+4x+3≥0⇔x−1(x+1)(x+3)≥0
Bảng xét dấu:
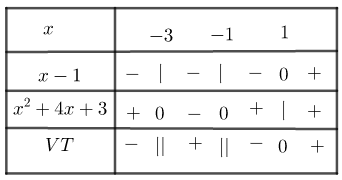
Từ bảng xét dấu ta có S=(−3;−1)∪[1;+∞)
Câu 2:
√2x2−5x+2≤2−x
Phương pháp giải:
√f(x)≤g(x)⇔{f(x)≥0g(x)≥0f(x)≤g2(x)
Lời giải chi tiết:
√2x2−5x+2≤2−x⇔{2−x≥02x2−5x+2≥02x2−5x+2≤x2−4x+4⇔{x≤2[x≥2x≤12x2−x−2≤0⇔{[x=2x≤12−1≤x≤2⇔[x=2−1≤x≤12⇒S={2}∪[−1;12]
Quảng cáo


















